How the game of blackjack derives a house percentage and what factors affect it.
In a card counting class I attended that was presented by Howard Grossman, he posed this question to the class; "If a player decided to play by the same rules as the dealer, namely hit sixteen or less and stand on seventeen and higher, why would the house have an advantage over this player?" Having read the answer in a book by John Scarne, I replied; "Because when the player busts, the dealer doesn’t go ahead and hit his hand to see if he will bust." This is called the "favorable bust percentage" and will occur approximately 8% of all hands. In other words, in 8% of all hands, the player busts and loses immediately, despite the fact that the dealer also busts or would bust, if he had completed the hand.
But the player that makes playing decisions identical to the dealer doesn’t suffer an 8% house percentage (HP). The reason being is that when the dealer gets blackjack, he only wins even-money but when the player gets blackjack, he wins 3 to 2. The 3 to 2 payoff for blackjack reduces the HP against the player by 2.5 % for a total of 5.5%. Some of the other game rules that affect HP are:
Dealer hits soft 17 + .2%
Re-split aces - .04%
Double down after split - .14%
Another factor that affects HP is the number of decks used:
Two decks + .32%
Four decks + .48%
Six decks + .54%
Eight decks + .58%
The last factor that affects HP is the playing decisions a player makes:
Proper standing on a stiff hand vs. a dealer upcard of 2-6 - 3.2%
Double down properly - 1.6%
Hitting soft hands properly - .3%
Proper splitting - .4%
This last factor is commonly referred to as "basic strategy" and quite simply is the mathematically proven correct way to decide hitting, double down and splitting decisions. If you add the total of all four of those percentages, you will discover that they total – 5.5%. So, in single deck game, correct basic strategy overcomes the house’s 5.5% HP and reduces it to zero and this is without the player even counting cards!
Of course, for many years, very few players played correct basic strategy and this is why single deck games continued to make money for the casinos. But with more and more educated players hitting the games, casinos decided they needed to make a rule change that would guarantee them a HP. This is why you now see casino only paying 6 to 5 for a player’s blackjack. This rule change added 1.20% to the casino’s HP and is generally considered sufficient not only to overcome a player playing perfect basic strategy but also a card counter as well.
In a card counting class I attended that was presented by Howard Grossman, he posed this question to the class; "If a player decided to play by the same rules as the dealer, namely hit sixteen or less and stand on seventeen and higher, why would the house have an advantage over this player?" Having read the answer in a book by John Scarne, I replied; "Because when the player busts, the dealer doesn’t go ahead and hit his hand to see if he will bust." This is called the "favorable bust percentage" and will occur approximately 8% of all hands. In other words, in 8% of all hands, the player busts and loses immediately, despite the fact that the dealer also busts or would bust, if he had completed the hand.
But the player that makes playing decisions identical to the dealer doesn’t suffer an 8% house percentage (HP). The reason being is that when the dealer gets blackjack, he only wins even-money but when the player gets blackjack, he wins 3 to 2. The 3 to 2 payoff for blackjack reduces the HP against the player by 2.5 % for a total of 5.5%. Some of the other game rules that affect HP are:
Dealer hits soft 17 + .2%
Re-split aces - .04%
Double down after split - .14%
Another factor that affects HP is the number of decks used:
Two decks + .32%
Four decks + .48%
Six decks + .54%
Eight decks + .58%
The last factor that affects HP is the playing decisions a player makes:
Proper standing on a stiff hand vs. a dealer upcard of 2-6 - 3.2%
Double down properly - 1.6%
Hitting soft hands properly - .3%
Proper splitting - .4%
This last factor is commonly referred to as "basic strategy" and quite simply is the mathematically proven correct way to decide hitting, double down and splitting decisions. If you add the total of all four of those percentages, you will discover that they total – 5.5%. So, in single deck game, correct basic strategy overcomes the house’s 5.5% HP and reduces it to zero and this is without the player even counting cards!
Of course, for many years, very few players played correct basic strategy and this is why single deck games continued to make money for the casinos. But with more and more educated players hitting the games, casinos decided they needed to make a rule change that would guarantee them a HP. This is why you now see casino only paying 6 to 5 for a player’s blackjack. This rule change added 1.20% to the casino’s HP and is generally considered sufficient not only to overcome a player playing perfect basic strategy but also a card counter as well.
- What you need to learn.
- Perfect knowledge of basic strategy.
- Perfect knowledge of a count system.
- True count conversion.
- Knowing the eighteen most important indices.
- What it takes for a counter to achieve a profit over the game.
The first step to mastering the game of blackjack is to completely memorize the basic strategy for the type of game you intend to play, or in our case, to watch. One of the things that has frustrated me, in my attempt to learn card counting over the years is my perception that no two "experts" can seem to agree on what the best basic strategy is. Part of this problem was mine; I didn’t realize that there were different strategies for different games. Part of this problem is with the people writing the books; despite the fact that they are all supposed to be following the same laws of mathematics, they keep coming up with different "mathematically correct" ways to play the same hand.
I intend to solve this problem by creating three rules of my own:
First: pick an expert and stick with him. I choose Bill Zender because what he teaches is more geared to the casino supervisor.
Second: if there are going to be differences, let us understand where they are most likely to occur. I have created a table addressing this problem and I think its creation is long overdue:
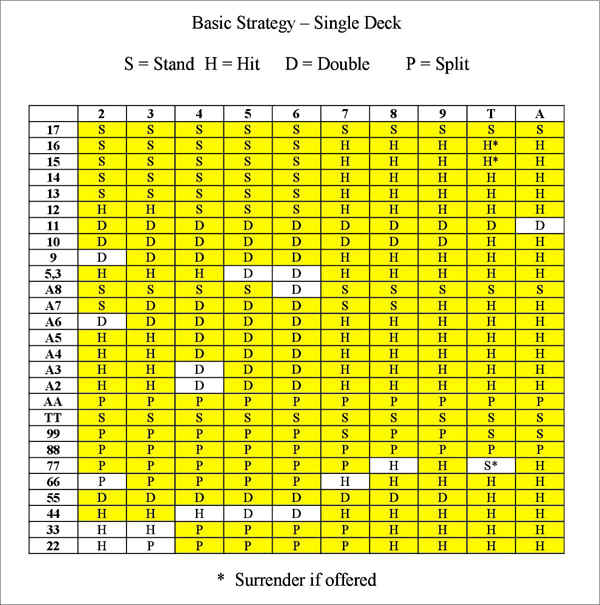
Here is the table for single deck without the option of doubling down after splitting. I have highlighted the decisions that should never change because of the number of decks, doubling rules or splitting rules. The yellow boxes represent the decisions of hitting, doubling down and splitting that should never change. For the sake of simplicity, I haven’t included the affected surrender decisions. If your boss gives you a basic strategy table that differs from one you have already memorized, if would be willing to bet that the differences are in the borderline decisions that aren’t highlighted on this chart.
After you have memorized a basic strategy chart, I hope that you will come back to this chart and look it over. I think by doing so, you will obtain a greater understanding of the game.
Third: as I alluded to in the beginning of this section, you should pick the basic strategy for the type of game you will most likely be watching.
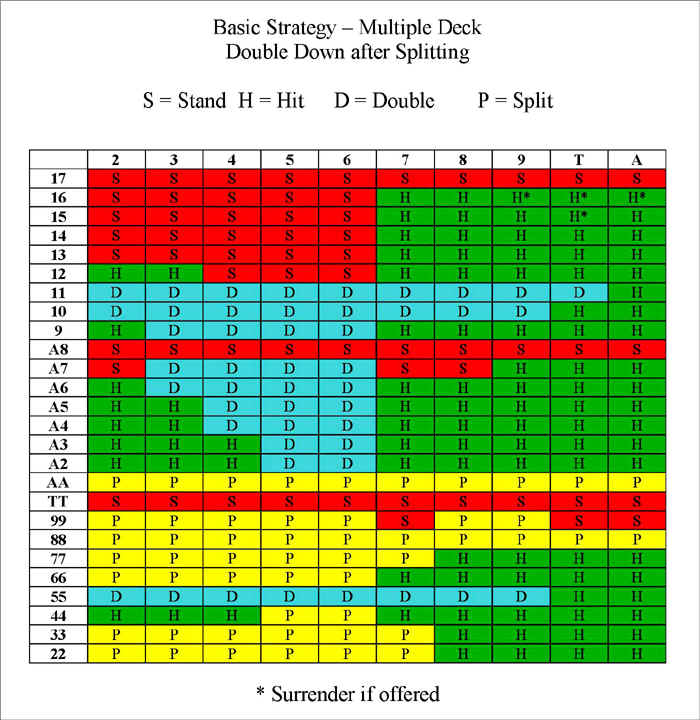
The basic strategy table that you will attack will be one of six:
After you have memorized a basic strategy chart, I hope that you will come back to this chart and look it over. I think by doing so, you will obtain a greater understanding of the game.
Third: as I alluded to in the beginning of this section, you should pick the basic strategy for the type of game you will most likely be watching.
The basic strategy table that you will attack will be one of six:
- Single deck.
- Single deck with double down after splitting option.
- Multiple deck.
- Multiple deck with double down after splitting option.
- Single deck, double down on 10 or 11 only.
- Multiple deck, double down on 10 or 11 only.
I have color-coded this chart to make it easier for you to follow:
Red = Stop = Stand
Green = Go = Hit
Blue = Double Down (I couldn’t think of a cute color code for double)
Yellow = Banana split (hee hee) = Split
Lets make some observations about this table and think up some mantras that will help us remember the rules:
Red = Stop = Stand
Green = Go = Hit
Blue = Double Down (I couldn’t think of a cute color code for double)
Yellow = Banana split (hee hee) = Split
Lets make some observations about this table and think up some mantras that will help us remember the rules:
- You will never hit hard 17 or higher.
- The only time you will hit a stiff against a stiff is when you have 12 against a 2 or 3.
- While most of us have learned to always double-down on 11, we now won’t when the upcard is an ace.
- Double on 10 except against a 10 or ace.
- Double on 9 against a 3 – 6 (notice that 3 + 6 = 9).
- Always stand on soft 19.
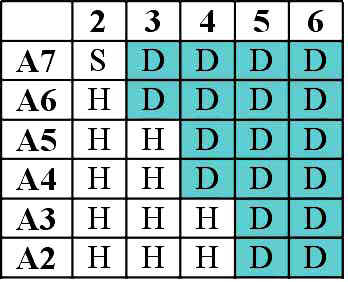
- On the soft totals, notice that the doubles form an upside-down step pattern like this:
So a soft total can only be doubled against an upcard of 5-6, 4-6 or 3-6; the higher the soft total, the greater the range that the doubling can occur. So, unless you are doubling, you are hitting, except:
The dreaded soft 18 (A7), which has a tendency to confuse us but isn’t really that difficult when you stop and think about the reason why we stand against a 2, 7 or 8. The dealer has more ways to make a hand with an upcard of 2, so it isn’t worth risking twice the bet. A soft 18 will win against a dealer 17 and push against a dealer 18, so why risk ruining that?
If surrender is offered: they should only do it when having 16 against a 9, 10 or ace or when having 15 against a 10. BTW, "early surrender" is when the player can surrender before the dealer checks for blackjack. "Late surrender" means the player can only surrender if the dealer doesn’t have blackjack.To memorize this chart you should first go over the previous observations while looking at the chart. You then should consider creating a blank table using MS WORD so you can print them out and practice completing them by hand.
I printed out a much smaller version of the table I have provided to you and taped it to a piece of cardboard the same size. I carry in my suit pocket so I can test myself by looking at the player’s hands and occasionally refer to it when I’m not sure if I’m right. The first step to protecting your games is to know when a player is playing perfect basic strategy. You need to have a complete mastery of basic strategy since you will need for it to become automatic, since things unfortunately get more difficult from here on.
The dreaded soft 18 (A7), which has a tendency to confuse us but isn’t really that difficult when you stop and think about the reason why we stand against a 2, 7 or 8. The dealer has more ways to make a hand with an upcard of 2, so it isn’t worth risking twice the bet. A soft 18 will win against a dealer 17 and push against a dealer 18, so why risk ruining that?
- Here is a rule that hasn’t changed since we were tourists; "Always split aces and eights."
- A player once asked a female dealer if he should have split his tens after he had done so and everyone at the table lost. Her reply was; "I suppose if you had a twenty inch dick, you’d cut that in half too!"
- "Split 9’s against all the stiffs, 8 and 9." We don’t split against a 7 since we assume we already have a winner.
- "Split 2’s, 3’s and 7’s against all the stiffs and a 7."
- "Split 6’s against all the stiffs."
- Treat a pair of fives just like it was a 10.
- "Split 4’s against 5 or 6 only."
If surrender is offered: they should only do it when having 16 against a 9, 10 or ace or when having 15 against a 10. BTW, "early surrender" is when the player can surrender before the dealer checks for blackjack. "Late surrender" means the player can only surrender if the dealer doesn’t have blackjack.To memorize this chart you should first go over the previous observations while looking at the chart. You then should consider creating a blank table using MS WORD so you can print them out and practice completing them by hand.
I printed out a much smaller version of the table I have provided to you and taped it to a piece of cardboard the same size. I carry in my suit pocket so I can test myself by looking at the player’s hands and occasionally refer to it when I’m not sure if I’m right. The first step to protecting your games is to know when a player is playing perfect basic strategy. You need to have a complete mastery of basic strategy since you will need for it to become automatic, since things unfortunately get more difficult from here on.
CARD COUNTING FOR FLOOR SUPERVISORS
Learning the Plus/Minus counting system.
Any method of card counting exists to accomplish three goals:
In the Plus/Minus count system; each card is assigned a value:
Learning the Plus/Minus counting system.
Any method of card counting exists to accomplish three goals:
- Tell the player when to bet more.
- Tell the player when to deviate from basic strategy.
- Tell the player when to take insurance.
In the Plus/Minus count system; each card is assigned a value:
The reason the 2 – 6 have positive values is because their removal from the deck is a good thing for the player. The dealer needs those small cards to make a good total when hitting stiff hands that the player doesn’t have to hit. The reason the tens and the aces have minus values is because their removal is bad for the player. The player gets paid 3 to 2 for a snapper and thus needs tens and aces in order to get them. The player also wants tens and aces for double downs. And the player wants tens in the deck so the dealer will draw them and bust his hand.
So, if the total of all exposed cards is a negative number, the remaining composition of the deck is favorable to the house. If the total of all exposed cards is a positive number, the remaining composition is favorable to the player.
To learn how to count cards using the Plus/Minus system is a three-step process:
1.) Turn over the cards in a deck, one at a time and state the value of the card. Don’t try to keep a running count, just identify the value of each card. When you can do this without hesitating, it is time to go to step two.
2.) Turn over the cards, one at a time and keep a running total. Since the Plus/Minus system is a "balanced system", after you turn over the last card, your running count should be zero. Get into the habit of not saying or thinking the word "plus" as this will slow you down. If the count isn’t minus, then it is plus. Don’t say or think the work "minus" either. Just use the word "my" instead. Do this exercise until you can do it without any mistakes 19 out of 20 times.
3.) Now you will begin to work on speed. Turn over the cards two at a time. If the total of the two cards adds to zero, then just drop them without saying anything. On a live game you will often see spreads of cards that add up to zero and you want to be able to develop the ability to recognize them. You will work up to the third exercise and do it until you can go through an entire deck in less than thirty seconds.
Converting a running count to a "true count."
A "true count" is the running count adjusted for the number of unseen decks and is the count the player will actually use to make decisions. During the seminar I mentioned earlier, that was given by Howard Grossman, I asked; "I don’t understand why any adjustments need to be made. If three aces have been played, why does it matter if they came from a single deck or a six-deck shoe? His response was; "Because your knowledge of three aces being played is diluted by the fact that there are many more cards left un-played, than if you were just counting down a single deck."
Computing the true count is easy. The only difficulty comes from the fact that on a live game it is just one more thing that you have to do. To convert a running count into a true count you merely divide the running count by the total number of unseen decks.
Example: The running count is +6 and there is three decks left. +6 / 3 = +2
Example: The running count is –4 and there is two decks left. –4 / 2 = -2.
As you are probably aware, whether the running count is a positive or negative number, converting it to a true count will never change the positive or negative aspect.
When there is less than one deck left unseen you can divide the running count by a fraction.
Example: The running count is +6 and there is 1/2 of a deck left. +6 / (1/2) = +12.
Example: The running count is –3 and there is 1/3 of a deck left. –3 / (1/3) = -9.
As long as the numerator of the fraction you are dividing by is "1", you merely have to multiply the running count by the denominator.
If the number of unseen decks is a whole number and a fraction, such as 2 1/2, I would just do the calculation twice and estimate the actual true count to be somewhere in the middle.
Example: The running count is +6 and there is 2 1/2 decks left.
+6 / 2 = +3 and +6 / 3 = +2 so I know the answer is greater than 2 and less than 3.
I have found the best way to practice my true count conversion is when I find myself in a section with the only live game being a shoe. I can then practice keeping a running count and converting it to a true count.
Learning the indices.
A card counter uses "indices" AKA "index numbers", "critical index numbers" or "matrix numbers" to tell him when to deviate from basic strategy. Learning the indices is where "we separate the men from the muchachos" and is absolutely necessary for the study of card counting. It is necessary for the card counter, since he won’t be able to gain an advantage from the house without knowing when to deviate from basic strategy. It is necessary for the supervisor since, seeing a player make a decision that goes against basic strategy, can give him a false sense of security if indices are not known.
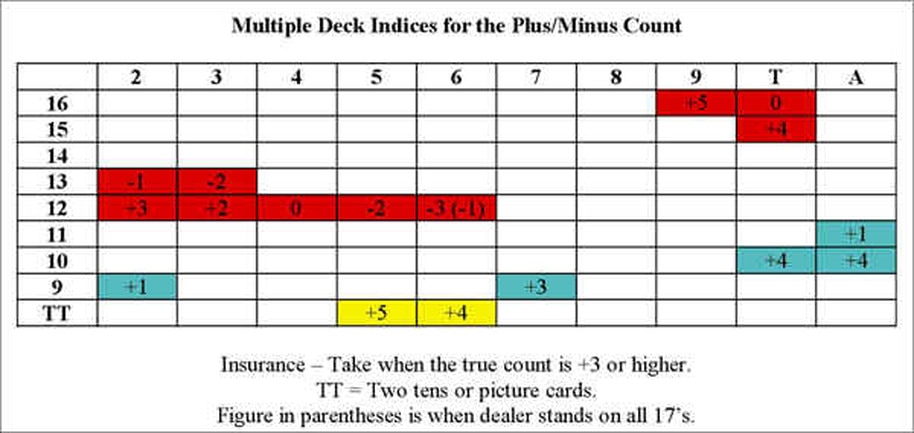
There are 140 indices for multi-deck games! The good news is there are only 18 that are considered the most important to learn and use and using them constitute 90% of the gain that can be achieved.
So, if the total of all exposed cards is a negative number, the remaining composition of the deck is favorable to the house. If the total of all exposed cards is a positive number, the remaining composition is favorable to the player.
To learn how to count cards using the Plus/Minus system is a three-step process:
1.) Turn over the cards in a deck, one at a time and state the value of the card. Don’t try to keep a running count, just identify the value of each card. When you can do this without hesitating, it is time to go to step two.
2.) Turn over the cards, one at a time and keep a running total. Since the Plus/Minus system is a "balanced system", after you turn over the last card, your running count should be zero. Get into the habit of not saying or thinking the word "plus" as this will slow you down. If the count isn’t minus, then it is plus. Don’t say or think the work "minus" either. Just use the word "my" instead. Do this exercise until you can do it without any mistakes 19 out of 20 times.
3.) Now you will begin to work on speed. Turn over the cards two at a time. If the total of the two cards adds to zero, then just drop them without saying anything. On a live game you will often see spreads of cards that add up to zero and you want to be able to develop the ability to recognize them. You will work up to the third exercise and do it until you can go through an entire deck in less than thirty seconds.
Converting a running count to a "true count."
A "true count" is the running count adjusted for the number of unseen decks and is the count the player will actually use to make decisions. During the seminar I mentioned earlier, that was given by Howard Grossman, I asked; "I don’t understand why any adjustments need to be made. If three aces have been played, why does it matter if they came from a single deck or a six-deck shoe? His response was; "Because your knowledge of three aces being played is diluted by the fact that there are many more cards left un-played, than if you were just counting down a single deck."
Computing the true count is easy. The only difficulty comes from the fact that on a live game it is just one more thing that you have to do. To convert a running count into a true count you merely divide the running count by the total number of unseen decks.
Example: The running count is +6 and there is three decks left. +6 / 3 = +2
Example: The running count is –4 and there is two decks left. –4 / 2 = -2.
As you are probably aware, whether the running count is a positive or negative number, converting it to a true count will never change the positive or negative aspect.
When there is less than one deck left unseen you can divide the running count by a fraction.
Example: The running count is +6 and there is 1/2 of a deck left. +6 / (1/2) = +12.
Example: The running count is –3 and there is 1/3 of a deck left. –3 / (1/3) = -9.
As long as the numerator of the fraction you are dividing by is "1", you merely have to multiply the running count by the denominator.
If the number of unseen decks is a whole number and a fraction, such as 2 1/2, I would just do the calculation twice and estimate the actual true count to be somewhere in the middle.
Example: The running count is +6 and there is 2 1/2 decks left.
+6 / 2 = +3 and +6 / 3 = +2 so I know the answer is greater than 2 and less than 3.
I have found the best way to practice my true count conversion is when I find myself in a section with the only live game being a shoe. I can then practice keeping a running count and converting it to a true count.
Learning the indices.
A card counter uses "indices" AKA "index numbers", "critical index numbers" or "matrix numbers" to tell him when to deviate from basic strategy. Learning the indices is where "we separate the men from the muchachos" and is absolutely necessary for the study of card counting. It is necessary for the card counter, since he won’t be able to gain an advantage from the house without knowing when to deviate from basic strategy. It is necessary for the supervisor since, seeing a player make a decision that goes against basic strategy, can give him a false sense of security if indices are not known.
There are 140 indices for multi-deck games! The good news is there are only 18 that are considered the most important to learn and use and using them constitute 90% of the gain that can be achieved.
Red – The player will stand when the true count is equal to or greater than the index number.
Blue – The player will double-down when the true count is equal to or greater than the index number.
Yellow – The player will split when the true count is equal to or greater than the index number.
These indices will be used in approximately 20% of all hands.
Don’t forget that a true count of –3 is less than a true count of –2.
You might be wondering why the index number for 16 against a 10 is zero. After all, if the true count is zero shouldn’t basic strategy be followed? The reason is: basic strategy has already taken into consideration the values of the cards exposed to get the player in that position.
The minus 1 value for 13 against a 2 is another confusing aspect, as it would seem that it is suggesting that you disregard basic strategy and "hit" 13 against a 2 when the true count is –1 or less. Remember, all indices for the hands of 12 – 16 indicate when the player will stand. If the true count is less than the index number, the player will hit.
To make sense of indices it is important to remember that if the true count is a negative number it indicates that 10’s and aces have been used. If the true count is positive, then it suggests that small cards have been used.
The first step to memorizing the indices is to confine your self to memorizing the plays that could potentially have an index number assigned to it. That way, if you are looking at a player with 15 against a dealer 9, you know that you don’t have to worry your pretty little head about what the index number is because there isn’t one. After memorizing the relevant plays, you then start to memorize the appropriate values to go with them. As with basic strategy, it is helpful to print out blank forms for the indices and practice completing them by hand. You can also practice them on the job, even if you haven’t been counting down the cards. For instance, if you see a player standing on 16 against a ten, you would be thinking that is only the correct play if the count is zero or higher.
And finally, don’t even think about working with indices until you have mastered basic strategy and the count system, as indices will be useless until you have.
What it takes for a card counter to achieve a profit over the game.
There are three characteristics that can be used to identify the card counter from the average gambler:
A player that sits through all counts and doesn’t leave a table will have to spread his bets to four units in order to gain his 1% advantage and win his one unit bet per hour.
A "Wonger" (named after its creator Stanford Wong, a Caucasian by the way) will "back count" a game or have team members playing on a game and signal him to play there, when the count is sufficiently high. A Wonger that "flat bets" (never varies the size of his bets) can still gain an almost 2% advantage over the house. However since he plays so few hands, he can only expect to win about 2/3 of a betting unit per hour.
Blue – The player will double-down when the true count is equal to or greater than the index number.
Yellow – The player will split when the true count is equal to or greater than the index number.
These indices will be used in approximately 20% of all hands.
Don’t forget that a true count of –3 is less than a true count of –2.
You might be wondering why the index number for 16 against a 10 is zero. After all, if the true count is zero shouldn’t basic strategy be followed? The reason is: basic strategy has already taken into consideration the values of the cards exposed to get the player in that position.
The minus 1 value for 13 against a 2 is another confusing aspect, as it would seem that it is suggesting that you disregard basic strategy and "hit" 13 against a 2 when the true count is –1 or less. Remember, all indices for the hands of 12 – 16 indicate when the player will stand. If the true count is less than the index number, the player will hit.
To make sense of indices it is important to remember that if the true count is a negative number it indicates that 10’s and aces have been used. If the true count is positive, then it suggests that small cards have been used.
The first step to memorizing the indices is to confine your self to memorizing the plays that could potentially have an index number assigned to it. That way, if you are looking at a player with 15 against a dealer 9, you know that you don’t have to worry your pretty little head about what the index number is because there isn’t one. After memorizing the relevant plays, you then start to memorize the appropriate values to go with them. As with basic strategy, it is helpful to print out blank forms for the indices and practice completing them by hand. You can also practice them on the job, even if you haven’t been counting down the cards. For instance, if you see a player standing on 16 against a ten, you would be thinking that is only the correct play if the count is zero or higher.
And finally, don’t even think about working with indices until you have mastered basic strategy and the count system, as indices will be useless until you have.
What it takes for a card counter to achieve a profit over the game.
There are three characteristics that can be used to identify the card counter from the average gambler:
- They must utilize a bet spread.
- They must deviate from basic strategy.
- They will only take insurance in high counts.
A player that sits through all counts and doesn’t leave a table will have to spread his bets to four units in order to gain his 1% advantage and win his one unit bet per hour.
A "Wonger" (named after its creator Stanford Wong, a Caucasian by the way) will "back count" a game or have team members playing on a game and signal him to play there, when the count is sufficiently high. A Wonger that "flat bets" (never varies the size of his bets) can still gain an almost 2% advantage over the house. However since he plays so few hands, he can only expect to win about 2/3 of a betting unit per hour.
How to identify a counter and report your suspicions.
It is much easier to prove that a player isn’t a card counter than to prove he is. It is virtually impossible to be sure a player is counting in under a half an hour. There are ways however, to prove that a player isn’t counting. If a player makes a large bet it can be assumed that the deck has a positive count. If the player fails to follow basic strategy or disregards basic strategy in a situation where indices say it should only be disregarded in a minus count, we know this player isn’t a counter.
Example: A player makes a large wager and hits a 10 and a 2 against a dealer 6. A true count of –3 would be required for the player to draw that card. Bottom line: either the player bet big into a minus count or he failed to make the correct play against a plus count. Therefore we can assume that he isn’t a counter.
Of course the possibility exists that a counter may intentionally make a bad basic strategy play when he has made a one-unit bet but he would have to be sure that you would notice it to make it worth risking even a small amount. Counters generally prefer to dissuade your suspicions by their acting performances rather than their play.
When it comes time to report your observations to your supervisor, you must be prepared to state the following facts:
What bet spread did the player use? Example: "He played one hand at $50 up to two hands at $200 each.
What was the count when he raised his bets? Example: "He bets $50 on minus counts and then $100 when the count hit +2 and then $200 each on two hands when the count is +4 or higher."
When did he deviate from basic strategy? Example: "He only hits 16 against a ten in a plus count, he split tens against a 5 when the count was +5 and he hit 13 against a 2 in minus counts."
How much do you calculate this player should win per hour? Example: "At his eight unit spread and the fact that he doesn’t change tables, I think he has a 1% advantage and should win just over one average bet per hour or about $125."
Don’t be disappointed if you boss decides not to back off the player. You have done your job and reported the facts as you saw them. What your boss decides to do with them is his prerogative.
It is much easier to prove that a player isn’t a card counter than to prove he is. It is virtually impossible to be sure a player is counting in under a half an hour. There are ways however, to prove that a player isn’t counting. If a player makes a large bet it can be assumed that the deck has a positive count. If the player fails to follow basic strategy or disregards basic strategy in a situation where indices say it should only be disregarded in a minus count, we know this player isn’t a counter.
Example: A player makes a large wager and hits a 10 and a 2 against a dealer 6. A true count of –3 would be required for the player to draw that card. Bottom line: either the player bet big into a minus count or he failed to make the correct play against a plus count. Therefore we can assume that he isn’t a counter.
Of course the possibility exists that a counter may intentionally make a bad basic strategy play when he has made a one-unit bet but he would have to be sure that you would notice it to make it worth risking even a small amount. Counters generally prefer to dissuade your suspicions by their acting performances rather than their play.
When it comes time to report your observations to your supervisor, you must be prepared to state the following facts:
What bet spread did the player use? Example: "He played one hand at $50 up to two hands at $200 each.
What was the count when he raised his bets? Example: "He bets $50 on minus counts and then $100 when the count hit +2 and then $200 each on two hands when the count is +4 or higher."
When did he deviate from basic strategy? Example: "He only hits 16 against a ten in a plus count, he split tens against a 5 when the count was +5 and he hit 13 against a 2 in minus counts."
How much do you calculate this player should win per hour? Example: "At his eight unit spread and the fact that he doesn’t change tables, I think he has a 1% advantage and should win just over one average bet per hour or about $125."
Don’t be disappointed if you boss decides not to back off the player. You have done your job and reported the facts as you saw them. What your boss decides to do with them is his prerogative.
Chapter 11
Techniques in Sitting Box
Techniques in Sitting Box
Site created and designed by
Scott Cameron
Las Vegas, Nevada
Scott Cameron
Las Vegas, Nevada
Email me [email protected]
Copyright 2020-2024
Last update 3/18/2024
Last update 3/18/2024
Visit my other website...